| CPC G06F 16/906 (2019.01) [G06F 16/9537 (2019.01)] | 10 Claims |
|
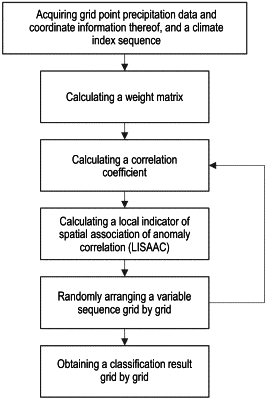
1. A teleconnection pattern-oriented spatial association clustering method, comprising following steps:
S1: acquiring coordinate information of a spatial grid in a research region, and calculating a spatial weight matrix according to the coordinate information;
S2: acquiring grid-scale precipitation data in the research region and large-scale climate indices in a same time range, and obtaining a precipitation and climate index time series;
S3: calculating a correlation coefficient r of a precipitation and the climate index grid by grid according to the precipitation and climate index time series, wherein the precipitation and climate index time series are obtained;
S4: calculating a local indicator of spatial association of anomaly correlation (LISAAC) grid by grid according to the correlation coefficient r and the spatial weight matrix;
S5: rearranging the precipitation and climate index time series in the step S2 to obtain a new precipitation and climate index time series, and repeating the steps S3 to S4 until a preset number n of iterations is reached;
S6: establishing a reference empirical distribution H according to n groups of random the LISAAC obtained in the step S4; and
S7: obtaining a classification result of each of grids at a specified significance level according to the LISAAC, the correlation coefficient r, and the reference empirical distribution H, wherein the LISAAC and the correlation coefficient r are observed.
|