US 11,898,094 B2
Systems and processes for improved drag reduction estimation and measurement
Nabijan Nizamidin, Houston, TX (US); Harold C. Linnemeyer, Sugar Land, TX (US); Timothy P. Theriot, The Woodlands, TX (US); Gojko Matovic, Cypress, TX (US); Seung Han, Katy, TX (US); Do Hoon Kim, Katy, TX (US); and Kerry K. Spilker, Houston, TX (US)
Assigned to Chevron U.S.A. INC.
Filed by Chevron U.S.A. INC., San Ramon, CA (US)
Filed on Nov. 25, 2020, as Appl. No. 17/104,514.
Application 17/104,514 is a continuation in part of application No. 16/697,426, filed on Nov. 27, 2019, granted, now 11,085,259.
Claims priority of provisional application 62/941,008, filed on Nov. 27, 2019.
Prior Publication US 2021/0198557 A1, Jul. 1, 2021
Int. Cl. C09K 8/62 (2006.01); C09K 8/60 (2006.01); G01N 11/04 (2006.01); E21B 43/26 (2006.01); E21B 47/00 (2012.01); G06F 30/28 (2020.01)
CPC C09K 8/62 (2013.01) [C09K 8/602 (2013.01); E21B 43/26 (2013.01); E21B 47/00 (2013.01); G01N 11/04 (2013.01); G06F 30/28 (2020.01); C09K 2208/08 (2013.01); C09K 2208/28 (2013.01)] 24 Claims
OG exemplary drawing
 
10. A process comprising:
preparing a fluid mixture for an oil field operation or a pipeline operation, the fluid mixture comprising a concentration of a drag reducing agent determined based on a drag reduction parameter ΔB for the fluid mixture; and
using the fluid mixture in the oil field operation or the pipeline operation;
wherein the process further comprises:
measuring one or more fluid properties of the fluid mixture in a laboratory, a flow loop, a portable apparatus, or any combination thereof;
determining a small-scale drag reduction parameter ΔB for the fluid mixture based on the one or more fluid properties of the fluid mixture measured in the laboratory or the flow loop; and
upscaling the small-scale drag reduction parameter ΔB for use in the oil field operation or the pipeline operation;
wherein an analytical model is used for upscaling the small-scale drag reduction parameter ΔB for use in the oil field operation or the pipeline operation;
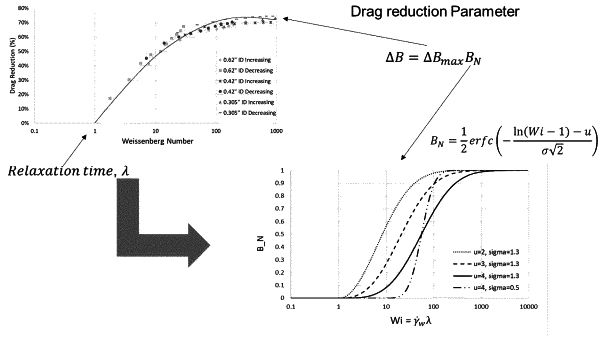
wherein the drag reduction parameter ΔB is defined by an equation:
ΔB=ΔBmaxBN
wherein
BN=0 for Wi<1 and
BN=CDF for 0<BN<1
wherein ΔBmax is maximum drag reduction at Wi>>1, BN is normalized drag reduction as a function of shear, Wi=γwλ is Weissenberg number, γw is the wall shear rate, λ is the longest relaxation time, and CDF is a cumulative distribution function.